407. Trapping Rain Water II

JIAKAOBO

LeetCode

感谢赞助!

• ㊗️
• 大家
• offer
• 多多！

Problem

Given an m x n integer matrix heightMap representing the height of each unit cell in a 2D elevation map, return the volume of water it can trap after raining.

Example 1:

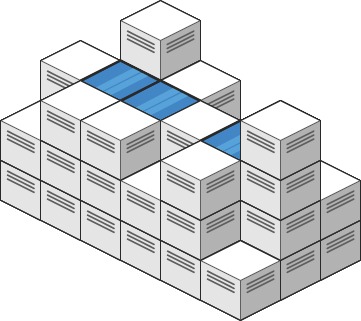
Input: heightMap = [[1,4,3,1,3,2],[3,2,1,3,2,4],[2,3,3,2,3,1]]
Output: 4
Explanation: After the rain, water is trapped between the blocks.
We have two small ponds 1 and 3 units trapped.
The total volume of water trapped is 4.

Example 2:

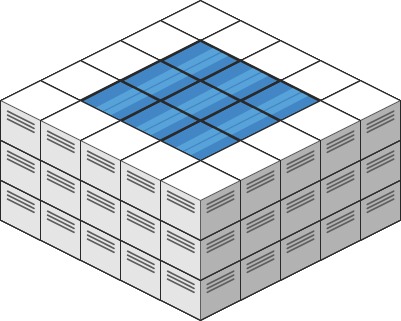
Input: heightMap = [[3,3,3,3,3],[3,2,2,2,3],[3,2,1,2,3],[3,2,2,2,3],[3,3,3,3,3]]
Output: 10

Code

class Solution {

    public class Cell {
        int row;
        int col;
        int height;
        public Cell(int row, int col, int height) {
            this.row = row;
            this.col = col;
            this.height = height;
        }
    }

    public int trapRainWater(int[][] heights) {
        if (heights == null || heights.length == 0 || heights[0].length == 0)
            return 0;

        PriorityQueue<Cell> queue = new PriorityQueue<>(1, new Comparator<Cell>(){
            public int compare(Cell a, Cell b) {
                return a.height - b.height;
            }
        });
        
        int m = heights.length;
        int n = heights[0].length;
        boolean[][] visited = new boolean[m][n];

        // Initially, add all the Cells which are on borders to the queue.
        for (int i = 0; i < m; i++) {
            visited[i][0] = true;
            visited[i][n - 1] = true;
            queue.offer(new Cell(i, 0, heights[i][0]));
            queue.offer(new Cell(i, n - 1, heights[i][n - 1]));
        }

        for (int i = 0; i < n; i++) {
            visited[0][i] = true;
            visited[m - 1][i] = true;
            queue.offer(new Cell(0, i, heights[0][i]));
            queue.offer(new Cell(m - 1, i, heights[m - 1][i]));
        }

        // from the borders, pick the shortest cell visited and check its neighbors:
        // if the neighbor is shorter, collect the water it can trap and update its height as its height plus the water trapped
       // add all its neighbors to the queue.
        int[][] dirs = new int[][]{{-1, 0}, {1, 0}, {0, -1}, {0, 1}};
        int res = 0;
        while (!queue.isEmpty()) {
            Cell cell = queue.poll();
            for (int[] dir : dirs) {
                int row = cell.row + dir[0];
                int col = cell.col + dir[1];
                if (row >= 0 && row < m && col >= 0 && col < n && !visited[row][col]) {
                    visited[row][col] = true;
                    res += Math.max(0, cell.height - heights[row][col]);
                    queue.offer(new Cell(row, col, Math.max(heights[row][col], cell.height)));
                }
            }
        }
        
        return res;
    }
}